ASSET ALLOCATION WITH TWO RISKY ASSETS

Covariance and Correlation

-Portfolio risk depends on the correlation between the returns of the assets in the portfolio
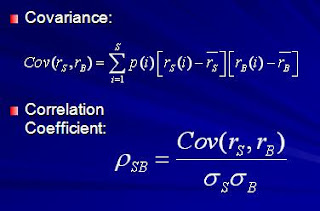
-Covariance and the correlation coefficient provide a measure of the returns on two assets to vary

Two Asset Portfolio Return – Stock and Bond

Covariance and Correlation Coefficient

Correlation Coefficients: Possible Values
Range of values for r 1,2
       -1.0 < r < 1.0
If r = 1.0, the securities would be perfectly positively correlated (meaning two assets move in the same direction)
If r = - 1.0, the securities would be perfectly negatively correlated (meaning two assets move in the opposite direction)

Two Asset Portfolio St Dev – Stock and Bond

In General, For an n-Security Portfolio:
rp = Weighted average of the
    n securities
sp2 = (Consider all pair-wise
          covariance measures)

Three Rules of Two-Risky-Asset Portfolios

Numerical Example: Bond and Stock Returns
Returns
    Bond = 6%    Stock = 10%
Standard Deviation
    Bond = 12%    Stock = 25%
Weights
    Bond = .5    Stock = .5
Correlation Coefficient
    (Bonds and Stock) = 0

Return = 8%
    .5(6) + .5 (10)

Standard Deviation = 13.87%
    [(.5)^2 (12)^2 + (.5)^2 (25)^2 + …
      2 (.5) (.5) (12) (25) (0)]^ ½
    [192.25]^ ½ = 13.87

Investment Opportunity Set for Stocks and Bonds

Investment Opportunity Set for Stocks and Bonds with Various Correlations